In order to fly north the plane should orient itself as if it were flying at an angle 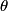west of north in still air, where

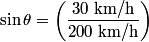When it does this, the velocity in the northward direction is

So, the time required to fly north is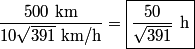

Therefore, answer (D) is correct.